[Set 237,169 on Mr. Square root]Zoom
[Japanese]
We will continue from where we ended in the last article, the article shows actual solutions to calculate Square root using abacus. Today's example is simple - basic Double-root method, root is 3-digits case. We require root reduction in the steps. Please check the Theory page for your reference.
Square root methods: Double-root method, Double-root alternative method, half-multiplication table method, half-multiplication table alternative method, multiplication-subtraction method, constant number method, etc.
Abacus steps to solve Square root of 237,169 (Answer is 487)
"1st group number" is the left most numbers in the 2-digits groups of the given number for square root calculation. Number of groups is the number of digits of the Square root.
237169 -> (23|71|69) : 23 is the 1st group number. The root digits is 3.
![]()
Step 1: Set 237169. 1st group is 23.
![]()
Step 2: Square number smaller than or equal to 23 is 16=4^2. 4 is the 1st root.
![]()
Step 3: Subtract 4^2 from the 1st group 23. 23-16=07
![]()
Step 4: Place 8 which is 2x of 1st root 4. This 8 is double root. Focus on 77.
![]()
Step 5: Divide 77 by 8. Answer=9 and this is 2nd root.
![]()
Step 6: Subtract 8x9=72 from 77. Place 05 on FG.
![]()
Step 7: You cannot subtract 9^2 (=81) from the 51 on GH, so the 2nd root 9 is over-root. Subtract 1 from 2nd root 9 then replace the 2nd root as 8, give back the double-root 8. Place 05+08=13 on FG.
![]()
Step 8: Focus on 2nd root 8 and 131 on FGH.
![]()
Step 9: Subtract 8^2 from 131 (FGH) and set the answer 067 to FGH.
![]()
Step 10: Focus on double-root 80 and 2nd root 8.
![]()
Step 11: Add 2 x 2nd root 8 (=16) to double-root 80. Place 2x8+80=96 on AB.
![]()
Step 12: Divide 676 on GHI by double-root 96.
![]()
Step 13: Answer=7 and this is 3rd root. Place 3rd root 7 on F. Place remainder 676-96x7=004 on GHI.
![]()
Step 14: Focus on 3rd root 7 and 3rd group 49 on IJ.
![]()
Step 15: Subtract 7^2 from 3rd group 49. Place 49-49=00 on IJ.
![]()
Step 16: Square root of 237169 is 487.
![]()
Final state: Answer 487
Abacus state transition. (Click to Zoom)
![]()
Next article is also about Double-root method.
Related articles:
How to solve Cube root of 1729.03 using abacus? (Feynman v.s. Abacus man)
http://blog.goo.ne.jp/ktonegaw/e/cff5d6e7ecaa07230b9cc7af10b23aed
Please place your mouse on the buttons and click one by one. These are blog ranking sites.
![にほんブログ村 科学ブログ 物理学へ]()
![人気ブログランキングへ]()
![]()
[Japanese]
We will continue from where we ended in the last article, the article shows actual solutions to calculate Square root using abacus. Today's example is simple - basic Double-root method, root is 3-digits case. We require root reduction in the steps. Please check the Theory page for your reference.
Square root methods: Double-root method, Double-root alternative method, half-multiplication table method, half-multiplication table alternative method, multiplication-subtraction method, constant number method, etc.
Abacus steps to solve Square root of 237,169 (Answer is 487)
"1st group number" is the left most numbers in the 2-digits groups of the given number for square root calculation. Number of groups is the number of digits of the Square root.
237169 -> (23|71|69) : 23 is the 1st group number. The root digits is 3.
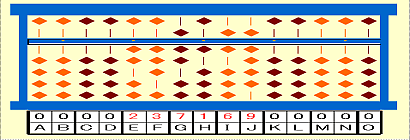
Step 1: Set 237169. 1st group is 23.
Step 2: Square number smaller than or equal to 23 is 16=4^2. 4 is the 1st root.
Step 3: Subtract 4^2 from the 1st group 23. 23-16=07
Step 4: Place 8 which is 2x of 1st root 4. This 8 is double root. Focus on 77.
Step 5: Divide 77 by 8. Answer=9 and this is 2nd root.
Step 6: Subtract 8x9=72 from 77. Place 05 on FG.
Step 7: You cannot subtract 9^2 (=81) from the 51 on GH, so the 2nd root 9 is over-root. Subtract 1 from 2nd root 9 then replace the 2nd root as 8, give back the double-root 8. Place 05+08=13 on FG.
Step 8: Focus on 2nd root 8 and 131 on FGH.
Step 9: Subtract 8^2 from 131 (FGH) and set the answer 067 to FGH.
Step 10: Focus on double-root 80 and 2nd root 8.
Step 11: Add 2 x 2nd root 8 (=16) to double-root 80. Place 2x8+80=96 on AB.
Step 12: Divide 676 on GHI by double-root 96.
Step 13: Answer=7 and this is 3rd root. Place 3rd root 7 on F. Place remainder 676-96x7=004 on GHI.
Step 14: Focus on 3rd root 7 and 3rd group 49 on IJ.
Step 15: Subtract 7^2 from 3rd group 49. Place 49-49=00 on IJ.
Step 16: Square root of 237169 is 487.
Final state: Answer 487
Abacus state transition. (Click to Zoom)
Next article is also about Double-root method.
Related articles:
How to solve Cube root of 1729.03 using abacus? (Feynman v.s. Abacus man)
http://blog.goo.ne.jp/ktonegaw/e/cff5d6e7ecaa07230b9cc7af10b23aed
Please place your mouse on the buttons and click one by one. These are blog ranking sites.
